we introduced circular motion and derived a formula which describes the linear
velocity of an object moving on a circular path at a constant angular velocity. One of the goals of
this section is describe the position of such an object. To that end, consider an angle θ in standard
position and let P denote the point where the terminal side of θ intersects the Unit Circle. By
associating the point P with the angle θ, we are assigning a position on the Unit Circle to the angle
θ. The x-coordinate of P is called the cosine of θ, written cos(θ), while the y-coordinate of P is
called the sine of θ, written sin(θ).1 The reader is encouraged to verify that these rules used to
match an angle with its cosine and sine do, in fact, satisfy the definition of a function. That is, for
each angle θ, there is only one associated value of cos(θ) and only one associated value of sin(θ).
Example:
Find the cosine and sine of the following angles.
1. θ = 270◦ 2. θ = −Ï€ 3. θ = 45◦ 4. θ =
Ï€
6
5. θ = 60◦
Solution.
1. To find cos (270◦
) and sin (270◦
), we plot the angle θ = 270◦
in standard position and find
the point on the terminal side of θ which lies on the Unit Circle. Since 270◦
represents 3
4
of a
counter-clockwise revolution, the terminal side of θ lies along the negative y-axis. Hence, the
point we seek is (0, −1) so that cos (270◦
) = 0 and sin (270◦
) = −1.
2. The angle θ = −Ï€ represents one half of a clockwise revolution so its terminal side lies on the
negative x-axis. The point on the Unit Circle that lies on the negative x-axis is (−1, 0) which
means cos(−Ï€) = −1 and sin(−Ï€) = 0.
Theorem 10.1. The Pythagorean Identity: For any angle θ, cos2
(θ) + sin2
(θ) = 1.
The moniker ‘Pythagorean’ brings to mind the Pythagorean Theorem, from which both the Distance
Formula and the equation for a circle are ultimately derived.5 The word ‘Identity’ reminds us that,
regardless of the angle θ, the equation in Theorem 10.1 is always true. If one of cos(θ) or sin(θ) is
known, Theorem 10.1 can be used to determine the other, up to a (±) sign. If, in addition, we know
where the terminal side of θ lies when in standard position, then we can remove the ambiguity of
the (±) and completely determine the missing value as the next example illustrates.
Example 10.2.2. Using the given information about θ, find the indicated value.
1. If θ is a Quadrant II angle with sin(θ) = 3/5
, find cos(θ).
2. If π < θ < 3π
2 with cos(θ) = −
√5/5
, find sin(θ).
3. If sin(θ) = 1, find cos(θ).
Solution.
1. When we substitute sin(θ) = 3/5
into The Pythagorean Identity, cos2
(θ) + sin2
(θ) = 1, we
obtain cos2
(θ) + 9/25 = 1. Solving, we find cos(θ) = ±
4
5
. Since θ is a Quadrant II angle, its
terminal side, when plotted in standard position, lies in Quadrant II. Since the x-coordinates
are negative in Quadrant II, cos(θ) is too. Hence, cos(θ) = −
4/5
.
2. Substituting cos(θ) = −
√5/5
into cos2
(θ) + sin2
(θ) = 1 gives sin(θ) = ± √2/5
= ±
2
√5/5
. Since we
are given that π < θ < 3π
2
, we know θ is a Quadrant III angle. Hence both its sine and cosine
are negative and we conclude sin(θ) = −
2
√
5
5
.
3. When we substitute sin(θ) = 1 into cos2
(θ) + sin2
(θ) = 1, we find cos(θ) = 0.
Another tool which helps immensely in determining cosines and sines of angles is the symmetry
inherent in the Unit Circle. Suppose, for instance, we wish to know the cosine and sine of θ =
5Ï€
6
.
We plot θ in standard position below and, as usual, let P(x, y) denote the point on the terminal
side of θ which lies on the Unit Circle. Note that the terminal side of θ lies π
6
radians short of one
half revolution. In Example 10.2.1, we determined that cos
Ï€
6
=
√3/2
and sin
Ï€
6
= 1/2 .
Theorem 10.2. Reference Angle Theorem. Suppose α is the reference angle for θ. Then
cos(θ) = ± cos(α) and sin(θ) = ± sin(α), where the choice of the (±) depends on the quadrant
in which the terminal side of θ lies.
Sine, Cosine and Tangent
Sine, Cosine and Tangent (often shortened to sin, cos and tan) are each a ratio of sides of a right angled triangle:
For a given angle θ each ratio stays the same
no matter how big or small the triangle is
To calculate them:
Divide the length of one side by another side
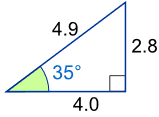
Example: What is the sine of 35°?
Using this triangle (lengths are only to one decimal place):
| sin(35°) | = OppositeHypotenuse |
| = 2.84.9 |
| = 0.57... |
| |
| cos(35°) | = AdjacentHypotenuse |
| = 4.04.9 |
| = 0.82... |
| |
| tan(35°) | = OppositeAdjacent |
| = 2.84.0 |
| = 0.70... |
Good calculators have sin, cos and tan on them, to make it easy for you. Just put in the angle and press the button.
But you still need to remember what they mean!
In picture form:
Sohcahtoa
How to remember? Think "Sohcahtoa"!
It works like this:
Soh...
|
Sine = Opposite / Hypotenuse
|
...cah...
|
Cosine = Adjacent / Hypotenuse
|
...toa
|
Tangent = Opposite / Adjacent
|
Less Common Functions
To complete the picture, there are 3 other functions where we divide one side by another, but they are not so commonly used.
They are equal to 1 divided by cos, 1 divided by sin, and 1 divided by tan:
Secant Function:
| | sec(θ) = HypotenuseAdjacent | | (=1/cos) |
Cosecant Function:
| | csc(θ) = HypotenuseOpposite | | (=1/sin) |
Cotangent Function:
| | cot(θ) = AdjacentOpposite | | (=1/tan) |
3. Parallel lines are straight lines which lie in the same plane and do not intersecr no matter how long they are extended.
and
): A number, 3.141592..., equal to (the circumference) / (the diameter) of any circle.
